The Linear and Quadratic Systems Worksheet presents a comprehensive exploration of these fundamental mathematical concepts, guiding learners through a journey of understanding and application. This worksheet serves as an invaluable resource for students, educators, and anyone seeking to enhance their knowledge of linear and quadratic systems.

As we embark on this intellectual endeavor, we will unravel the intricacies of linear systems, delve into the depths of quadratic systems, and uncover the methods for solving systems of linear and quadratic equations. Through real-world applications and practice problems, this worksheet fosters a deep comprehension of these essential mathematical concepts.

Linear Systems: Linear And Quadratic Systems Worksheet

Linear systems consist of equations with variables that have a degree of 1. In other words, the variables are not squared or raised to any power other than 1. Linear systems can be represented in the form Ax + By = C, where A, B, and C are constants and x and y are variables.

Examples of Linear Systems

• 2x + 3y = 5
• x – y = 2
• -3x + 2y = 1

Solution Methods for Linear Systems

System of Equations	Solution Method	Solution
2x + 3y = 5x y = 2	Substitution	x = 1, y = 1
x y = 2-3x + 2y = 1	Elimination	x = 3, y = 1

Quadratic Systems

Quadratic systems consist of equations with variables that have a degree of 2. In other words, at least one of the variables is squared. Quadratic systems can be represented in the form ax² + bx + c = 0, where a, b, and c are constants and x is a variable.

Methods for Solving Quadratic Systems, Linear and quadratic systems worksheet

Method	Steps
Factoring	Factor the quadratic equation into two binomials. Set each binomial equal to zero and solve for the variable.
Completing the Square	Add and subtract the square of half the coefficient of the x-term. Factor the resulting expression as a perfect square trinomial. Solve for the variable.
Quadratic Formula	Use the quadratic formula: x = (-b ± √(b²- 4ac)) / 2a.

Systems of Linear and Quadratic Equations

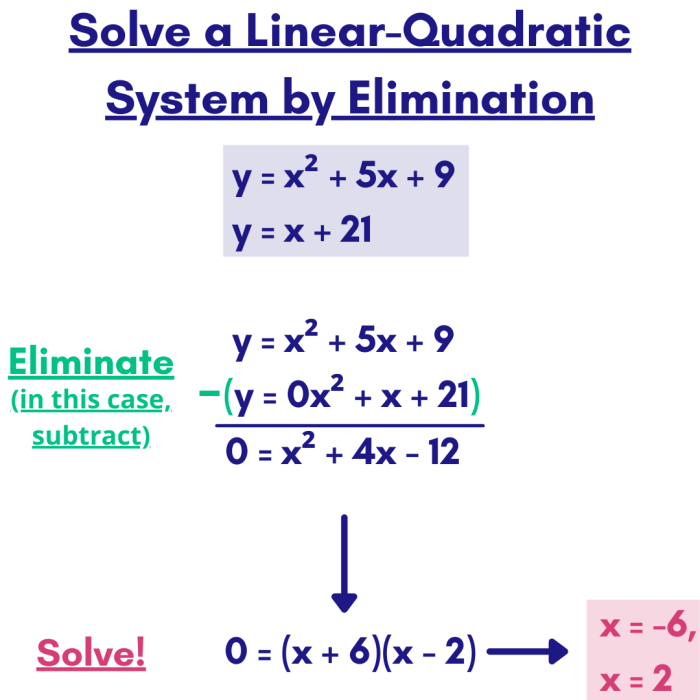
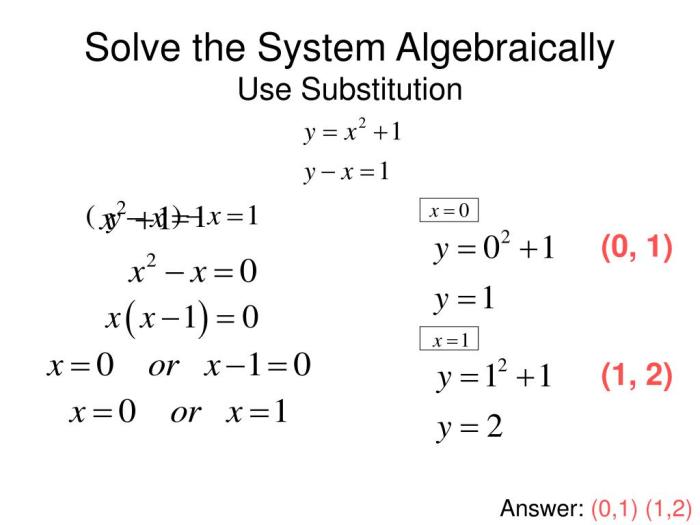
Systems of linear and quadratic equations consist of one linear equation and one quadratic equation. These systems can be solved by substitution or elimination.

Steps for Solving Systems of Linear and Quadratic Equations

• Solve the linear equation for one of the variables.
• Substitute the expression for the variable from the linear equation into the quadratic equation.
• Solve the resulting quadratic equation for the other variable.
• Substitute the value of the variable from the quadratic equation back into the linear equation to find the value of the first variable.

Applications of Linear and Quadratic Systems

Linear and quadratic systems have many real-world applications, including:

Application	Corresponding System of Equations	Solution
Projectile Motion	v = u + ats = ut + 1/2at²	v = final velocity u = initial velocity a = acceleration t = time s = distance
Finance	I = PrtA = P(1 + r/n)^(nt)	I = interest P = principal r = interest rate t = time n = number of compounding periods per year A = future value

Practice Problems

Problem	Solution Method	Solution
Solve the system of equations:2x + 3y = 5x y = 2	Substitution	x = 1, y = 1
Solve the quadratic equation:x² 5x + 6 = 0	Factoring	x = 2, x = 3
Solve the system of equations:y = x + 1 x² + y² = 25	Substitution	x = 2, y = 3x = 2, y = 1

Essential Questionnaire

What is the primary objective of this worksheet?

The Linear and Quadratic Systems Worksheet aims to provide a thorough understanding of linear and quadratic systems, their solution methods, and their applications in real-world scenarios.

What topics are covered in this worksheet?

This worksheet encompasses the concepts of linear systems, quadratic systems, systems of linear and quadratic equations, and their practical applications.

Is this worksheet suitable for all levels of learners?

The worksheet is designed to cater to a wide range of learners, from beginners seeking a foundational understanding to advanced students looking to reinforce their knowledge.